I have no idea what to do.
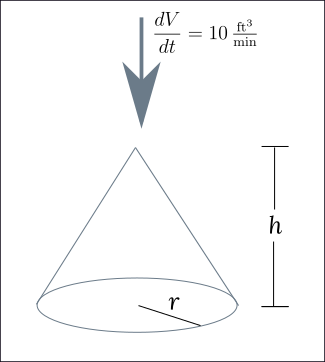
Gravel is being dumped from a conveyor belt at a rate of 10 cubic feet per minute. It forms a pile in the shape of a right circular cone whose base diameter and height are always equal. How fast is the height of the pile increasing when the pile is 14 feet high?
Recall that the volume of a right circular cone with height h and radius of the base r is given by
V=1/3πr^2h
Hi MattC, welcome to the Matheno community.
Good job on identifying this problem as a “Related Rates” type. We have a 4-step method for solving related rates problem that can be found here.
The example we present is very similar, the only differences are that the volume in your problem is increasing at 10 \text{ ft}^3/\text{ min} and the relationship between the height and radius is r=\dfrac{h}{2}. You may want to make sure you state your answer in the correct units.
thanks a lot that helped
1 Like